


Is the Universe spatially closed or open? Has it holes or handles, is it connected or not ? Often neglected by cosmologists, the study of the topological classification of three-dimensional manifolds is likely to bring original answers to the question of the space extension. In the so-called " crumpled " universe models, the sky is the arena of a gigantic optical illusion, due a topological lens effect.
by Jean-Pierre Luminet
Research Director at CNRS
Astrophysicist at the Paris-Meudon Observatory.
General relativity upsets the concepts of time and space. The universe does not have a structure of immutable Euclidean space woven by an independent time; it is described as a space-time distorted by the presence of matter and energy. As a manifestation of the curvature of space-time, gravitation dictates the trajectories of particles and light rays, compelled to marry contours of a non-Euclidean four-dimensional geometry.
The basic equations of relativity describe the way the material contents of the universe determines the background geometry of space-time. In this way, the theory makes it possible to describe the universe as a whole according to plausible cosmological models. Among the solutions, only a few correctly describe the universe, providing a theoretically consistent explanation of astronomical observations.
In 1917 Einstein built the first universe model based on his theory of relativity. Its great breakthrough was to propose a new approach of the question of space. Indeed, the non-Euclidean geometry makes it possible to describe a space which is both finite and unbounded: the hypersphere. Einstein thus offered, for the first time in the history of cosmology, a model of a finite universe free from any " edge " paradox.
The advocates of a finite world butted a long time against
a fundamental difficulty. It seemed essential to imagine
a center and a border to the world, but Archytas of Tarente, a pythagorician
from Vth
century, raised a paradox aiming at showing the nonsense of the idea
of a physical edge of the world. Its argument knew a considerable
fortune in all the debates on space : " If I am at the end of
the sky, can I lengthen the hand or a stick? It is absurd to think
that I cannot ; and if I can , what is beyond is either a body,
or space. We can thus go beyond that still, and so on. And if there
is always a new space towards which one can tighten the stick, that
implies an extension without limits clearly ".
If" what is beyond the world" always formed part of the world,
the world cannot logically be limited without there being paradox! It
was necessary to await the development of the non-Euclidean geometries
in XIXth century to solve the controversy. These geometries make it
possible to conceive a space as finite but without edge (just
like, in two dimensions, the surface of a sphere). This design is not so natural
and confusion is still found today in a number of minds; when, for
example, a lecturer describes to a popular audience the expansion of the universe, it is
often seen raising the question: in what the universe does inflate?
The question itself is a semantic non-sense,
since there is no space apart from itself ! But to
really understand this, it should be adopted a non-Euclidean mental
framework.
Besides the conceptual revolution resulting from relativity, observational progress led Hubble to announce, in 1929, that the other galaxies move away systematically from ours, with velocities proportional to their distance. The Einstein model thus had to be abandoned because it described a static universe, and replaced by dynamical universe models, independently discovered by the Russian Alexandre Friedmann and the Belgian George Lemaître.
The question of the extension of space is perfectly well put within the framework of the Friedmann-Lemaître models, called more commonly "big-bang models". These ones assume that the universe has the same properties everywhere (space is known as " homogeneous and isotropic "). The geometrical properties of space are of two kinds only: the curvature, constant in space when matter is uniformly distributed, and the topology. Regarding the curvature, three families of spaces can be considered: Euclidean space (zero curvature), spherical space (positive curvature) and hyperbolic space (negative curvature). Spherical space is, in all the cases, finite (it is one of the reasons for which Einstein, in the spirit of Parmenides, choose it initially). For spaces belonging to the two other families, the finite or infinite character depends on topology. In the simplest versions however (simply-connected topologies), they are infinite.
The cosmologists generally neglect the topological aspect to consider only the curvature properties. This simplification is dramatic as for the problem of infinite space since, in such a case, the dilemma finite/infinite is brought back to know the sign of the space curvature only.
General relativity indicates how to calculate this curvature. Its value depends on the average density of matter-energy it contains, as well as a parameter Lambda, called the cosmological constant. Generally, a second simplification is introduced, that to suppose a vanishing Lambda. Then, the finite/infinite character of space does not depend any more but on the average matter-energy density: according to whether it is higher or lower than a certain "critical value ", 10^(-29) g/cm3, the curvature is positive or negative, and space is finite or infinite. What are the observational data? They indicate an average density approximately ten times lower than the critical value. Apparently, if the topological complications and the cosmological constant are neglected, space would be thus infinite. In fact, the actual value is only a lower limit. It would be non-sense to believe that we see all the matter in the universe. Various reasons suggest that, in addition to visible matter, great quantities of dark matter exist, sufficiently perhaps so that the true density of the universe reaches exactly the critical value. In this case, the universe would marginally remain open in space and time. This is the Euclidean model, first proposed by Einstein and de Sitter in 1931, and which keeps still today the favours of many cosmologists, without decisive argument to justify it (if not... an aesthetic feeling)

|
Is the Universe closed or open ?
In the Friedmann-Lemaître cosmological models with vanishing cosmological constant, the curvature is directly related to the average energy density: the curvature is positive (spherical space) when the density is higher than the critical value, zero (Euclidean space) if the density is equal to the critical value, and negative (hyperbolic space) if density is lower. The curvature thus dictates only the time evolution: the universe is (temporally) closed in the spherical case, (temporally) open in the Euclidean and hyperbolic cases. The Einstein-de Sitter flat model (that some cosmologists estimate favoured by inflationary models of the early universe) corresponds to the diagram of the middle. If moreover the simplest topology is assumed, the curvature dictates also the finite or infinite character of space: finite in the spherical case, infinite in the Euclidean and hyperbolic cases. With these two (unjustified) simplifications, there is strict equivalence between time finiteness /infiniteness and space finiteness / infiniteness. In the Friedmann-Lemaître models with non zero cosmological constant, the curvature is related to the matter density and the cosmological constant. There is no more direct link between the curvature and the cosmic dynamics : the universe can be spherical but temporally open. If, moreover, topology is not the simplest one, there is no more correspondence between time finiteness / infiniteness and space finiteness / infiniteness.
|
If the paradox of the edge made obstacle to the concept of finite space, the " dark night paradox " made obstacle to space infinity. The darkness of the night indeed hides a mystery involving the cosmos as a whole, its extension and its history. It is stated like follows: if space is infinite and uniformly filled with eternal stars, in any direction which one looks at one must end up finding a star on the line of sight. In other words, the sky background should be a radiant tapestry, continuously made up of stars, not leaving any place to the dark. Why isn't it thus? The question, put as of XVIIth century by Kepler (and later by Olbers), raised tens of explanations and models. The American writer Edgar Poe provided the first satisfactory answer. In a premonitory text entitled Eureka, Poe explained why the darkness of the night rested on the finitude of cosmic time. Indeed, as he pointed out, the light can propagate only at finite speed. However, in a non-eternal universe, the stars did not always exist. We can thus receive their light only if this one had time to reach us, i.e. if the stars which emitted it were sufficiently close. Thus, the sky is not uniformly brilliant because the stars (not necessarily the entire universe) have existed only for a finite time. By understanding how the night darkness privided to us a deep teaching about the time finiteness of the world, Poe anticipated by several decades the big-bang relativistic models.

|
The cosmic microwave background radiation
Since the universe has not existed (at least in a state allowing the existence of
stars) for more than a few billion years, the sky background is hardly brilliant. It emits a weak gleam,
unperceivable to our eyes, but that radiotelescopes can collect. Discovered
in 1965, it is the vestige of
dazzling primitive fire cooled by fifteen billion years of time travel.
|
The questions related to the global shape of space and, in particular, its finite or infinite extension, cannot be fully answered by general relativity (a local physical theory), but by topology (a global mathematical theory).
Nothing obliges space to have the simplest topology (known as " simply-connected ") because general relativity does not impose any constraint on the global properties of space-time. Many topological "alternatives" of three-dimensional spaces can thus be used to build relevant universe models, i.e. both compatible with relativity and observations.
Thanks to " multi-connected " topologies, it becomes possible to consider universe models where space is finite whatever its curvature, even if the matter density and the cosmological constant are very low.
Historically, W. de Sitter pointed out in 1917 to Einstein that his static and spherical universe model could put up with a different topology, namely that of projective space. The difference was not very large because these two alternatives are finite. The outstanding article by Friedmann, in 1922, makes mention of a finite Euclidean space form (normally infinite). Einstein remained unaware of that since, in 1931, he published with de Sitter an article where they selected the infinite Euclidean universe model. Only in 1958, Lemaître mentioned the existence of compact hyperbolic spaces, also suitable for application to big-bang models. In spite of that, the subject of cosmic topology always remained confidential and widely ignored by the community of cosmologists.
In addition to the interest of "compactifying" spaces, the multi-connected models cause many surprises by creating an "illusion of the infinity". Let us see why. To build multi-connected spaces, mathematics teach us that one can start from one of the three types of " ordinary " (simply connected) spaces. Then, identification between some points change the shape of space and makes it multi-connected. From this one can build universe models where space is finite (although the curvature can be negative or zero) and of a really small volume. They are called "small universes". The simplest example is when our space would be a hypertorus having a radius lower than five billion light-years. In this case, the light rays would have had time to turn three times "around" the universe. That would imply that each cosmic object (each galaxy for example) should appear according to as many "ghost" images, observable in various areas of the sky. The observed universe thus appears made up of the repetition of a same set of galaxies, although viewed at different look-back times.
It is not easy to check if we live or not in a small universe. The ghost images of each " real " galaxy would appear in different directions, with different luminosities, under different orientations, and at different evolutionary times. It would be practically impossible to recognize them like such! The universe could appear vast to us, " unfolded ", filled of billion galaxies, while it would actually be much smaller, " folded up " but containing only a small number of authentic objects. A gigantic cosmic optical illusion! Of course, the current observational data make it possible to eliminate the possibility of a too small universe... If not we would have already recognized, close to us, the multiple images of our own Galaxy! Various arguments of this kind, applied to some well-known cosmic objects (e.g. the closest galaxy clusters), make it possible to exclude a universe whose dimensions would be lower than a few hundreds of million light-years. However statistical studies on the distribution of galaxy clusters may reveal in the future the "crumpled" nature of space over a scale of a few billion light-years.

|
We see a sky filled with galaxies, but its aspect does not make it possible
to decide if the farthest galaxies are not ghost images of closer galaxies. The assumption of a
multi-connected Universe cannot be discarded: the Universe could appear vast
to us, " unfolded ", while it would be actually much smaller and "folded up".
|
Topology is the branch of geometry which classifies spaces according to
their global shape. By
definition, spaces belong to a same topological class if they can deduced from each other by continuous deformation,
i.e. without cutting nor tearing. In the case of two dimensional spaces, i.e. surfaces, the sphere, for
example, has the same topology as any ovoid closed surface. But the plane
has a different topology,
since no continuous deformation will give it the shape of a sphere.
For better visualizing what
is topology, start from the ordinary Euclidean plane. It is an infinite 2-dimensional layer (that
one generally imagines as embedded in ordinary 3 dimensional space). Let us
cut out a tape with infinite " length " but
finite width; then let us identify (i.e. restick) the two edges of this
tape: one gets a
cylinder, i.e. a surface whose topology differs from that of the initial
plane. Let us take another
infinite sheet and, this time, cut out it in rectangle.
Let us identify two by two the parallel edges. We obtain a closed (finite) surface.
It is a flat torus.
From a simple paper sheet we could thus define 3 surfaces with different topologies, pertaining to the
same family of locally flat surfaces.
During the XXth century, mathematicians stuck to the classification of three-dimensional spaces.
Like surfaces, 3-spaces can be arranged,
according to the sign of their curvature, into spherical, Euclidean
or hyperbolic types.
Then one counts the topological forms inside each one of these families. There are for
example 18 kinds of three-dimensional spaces with zero curvature. Simplest
is " ordinary "
infinite Euclidean space, the properties of which are teached at school,
but others space forms are closed and finite. It is for example the case of
the hypertorus, which generalizes
in three dimensions the case of the torus.
A hypertorus can be regarded as the interior of an ordinary cube, whose opposite faces
are identified two by two: while leaving by one, one returns immediately by
the opposite. Such a
space is finite.
In addition, there are a countable infinity of spaces
forms with positive curvature, all of them
closed, and an infinite number of spaces with negative curve, some closed
(finite), some open
(infinite).
To visualize them, one represents them by the interior of a polyhedron of which some
faces are identified two by two.

The five regular polyhedrons, already called upon by Plato for geometrizing the " elements " Earth, Water, Air, Fire, Quintessence, are used today to represent certain multi-connected spaces, on the condition of considering that the faces are identified by pairs according to specific geometrical transformations.

|
A compact hyperbolic space. The interior of a regular dodecahedron, whose pentagonal faces are identified ("stuck") by pairs, is a closed space of negative curve. Seen from inside, such a space would give the impression we live in a cellular space, paved ad infinitum by dodecahedrons deformed by optical illusions. Copyright 1990 by The Geometry Center, University of Minnesota.
|
Who wasn't fascinated by sets of mirrors? That it is about the Galerie des Glaces at the Chateau de Versailles or most modest Palais of the Ices of open attractions, each one is filled with wonder at the illusion generated by the phantom images. The mirrors conceal certain secrecies of infinity.
Everyone noted that to put mirrors on the walls of a room gives the illusion of a larger room.
Let us take a room filled with mirrors on its six walls (floor and ceiling included). If you penetrate in the room and light some candles, by the play of the multiple reflexions on the walls you have immediately the impression to see the infinity, as if you were suspended on the node of a bottomless well, ready to be swallowed in a direction or another with the least movement.
It could well be thus of cosmic space!
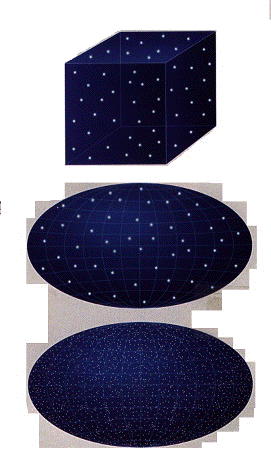
|
It may be that the topology of the universe is multiconnected, i.e. that space
resembles inside a room papered
with complicated mirrors. This multiconnexity would create additional
paths for the light rays which reach us from the remote galaxies. It would result a great
number of ghost images of these galaxies. The diagrams on the left result
from numerical simulations
of "crumpled" universes, carried out with my collaborators.
top diagram : space is a hypertorus, represented by the interior of a cube of 5 billion light-years size, whose opposite faces are identical. 50 galaxies are randomly distributed in space. middle diagram : positions, on a celestial planisphere, of the 50 " original " galaxies. bottom diagram : appearance of the sky taking account of the multiple
paths of light
rays. Each " real " galaxy generates about fifty "ghost" images.
It is impossible to distinguish the "real" images from the ghost images. If
one points out the
resemblance of this diagram to the appearance of the large scale structure
in the universe, one deduces that it is
quite possible that we live in a cosmic optical illusion, giving the impression
that space is immense, whereas real space is small and "crumpled".
|
It is clear that the concept of a small crumpled universe concerns Parmenidian aesthetics. This one took besides the step at the majority of modern physicists, who seek to eliminate infinite quantities from their theories. Space infinity is not the only infinity occurring in relativistic cosmology. The theory predicts configurations indeed where certain geometrical (e.g. curvature) and physical (e.g. energy density, temperature) quantities become infinite: gravitational singularities. Most known are the initial big-bang singularity, and the final singularity hidden at the bottom of a black hole. The physicists doubt that a theory predicting singularities can be correct. The fact is that general relativity is incomplete, since it does not take account of the principles of quantum mechanics. This last governs the evolution of microscopic world, in particular the field of elementary particles. Its essential characteristic is to give a " fuzzy " description of the phenomena, insofar as the events can be calculated only in terms of probabilities. However, the occurrence of singularities brings into play the structure of space-time at very small scale. There is a length (called Planck length, equal to 10^(-33) centimetre) representing the smallest dimension to which space-time can still be regarded as smooth. Below, even the texture of space-time would not be continuous any more but, just like the matter and the energy, formed of small grains. The gravitational infinities would be replaced by quantum fluctuations of space-time.
With " quantum cosmology ", a theory hardly outlined and promised to attractive developments, are profiled multiple, simultaneous and not-interacting bubble universes, differing from each other by their geometry, their topology, their fundamental constants of physics.
All these universes would be like the foam of a single Universe, a kind of infinite and eternal bubbling ocean, in perpetual transformation, called by the physicists " quantum vacuum ". With such a conception, Heraclitus' sons did not say their last word...
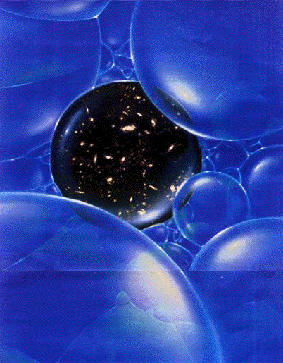
|
The Foam of Vacuum Quantum cosmology makes it possible to consider multiple universes, without interaction between them. Our observable universe would occupy a " bubble " born of the spontaneous fluctuations of the quantum vacuum, like many other bubbles.
Copyright : Manchu/Ciel et Espace
|
A hybrid creature given birth to by non-Euclidean geometry and relativistic gravitation, the
black hole offers two pretty problems of infinity: a false one and a true
one. A black hole results from
the gravitational collapse of a mass below some critical volume. Like the edge of a bottomless well
dug in the elastic fabric of space-time, its surface - called the event
horizon - marks the geometrical border of a
no return zone. For an external observer, the beats of a clock placed close to the black hole slow
down as the clock is closer to event horizon, until "freezing" when the clock
reaches the surface. All occurs
then as if time were indefinitely delayed. Consequently, the black hole by itself
is inobservable, because it belongs to the infinitely remote future of any observer.
This infinite time is only apparent because it can be
made finite in a correct representation (proper time).
The situation is quite
different with the interior of the black hole.
The general relativity theory predicts the existence of an inescapable singularity inside the black hole, where
the curvature of space and the density of matter become infinite.
A traveller exploring the surroundings of a black hole would be plunged in optical illusions. Misled by the infinite forgery related to the surface of the hole, it would never see the interior, unless plunging in person to discover there with its costs the infinite truth of the singularity!